Strike It Rich By Mining Your Debt: There’s Gold In Them Thar Loans!

The topic of debt usually gets pretty rough treatment in the personal finance blogging community. And for good reason – if not handled with care, it can be as dangerous as a short-fused stick o’ dynamite.
But if you’re already in debt and your money situation feels as cramped as a claustrophobic miner trapped deep underground, you’re already acutely aware of how dangerous debt can be. So let’s pivot and instead explore a far more encouraging aspect of debt – it’s hidden wealth potential.
Yep, you read that right. If you’re in debt you are sitting on a potential mother lode of riches. So without further ado, let’s stake out your debt claim and discover how you can strike it rich by mining your debt.
Table of Contents
In Pursuit Of Profit – The ’49er Mentality
The 1848 discovery of gold at Sutter’s Mill triggered the famous California Gold Rush. In all, over 300,000 aspiring miners left home behind in pursuit of profit and riches in the California gold fields. Those who did so would later become known as “49ers”, a reference to the year many arrived in the state (1849).
Just like a ’49er, you should be in pursuit of profit – in the gold fields of your monthly budget. At this point in the Master Your Money series, we’re working on using the net profit of your monthly budget to identify and fund savings goals in support of your ideal future lifestyle. The bigger the profit, the faster you will achieve financial freedom!
But if your net profit currently seems far too low for you to ever be able to afford the items on your financial freedom shopping list, you might be somewhat discouraged. If that’s the case, chin up! Because like a mining boomtown that simply springs up overnight, you can grow the monthly net profit of your household budget explosively by mining your debt.
The Greatest Mining Boomtown In American History
The year was 1859. Virginia City, Nevada was born overnight after the discovery of the Comstock Lode, the first major silver deposit identified in the U.S.
In a span of just four years, Virginia City grew from a mere loose collection of tents to a bustling industrial destination with a burgeoning population of 15,000. By the mid-1870’s, it had grown to 25,000. Its growth was unrivaled by that of any other mining boomtowns in history.
Nevada obtained territorial status in 1861 and statehood in 1864 thanks in part to the booming wealth and population of Virginia City. Within just a few years, Nevada’s statehood played a key role in the passage of the 13th & 15th Amendments to the U.S. Constitution.
The Richest City In America
By the 1870’s, more than half of all precious metals produced in the U.S. originated from Nevada, and Virginia City was known as the richest city in America. Much of the profits from its mines were used to invest in the development of San Francisco real estate, building the metropolis that we know today.
Silver was actually demonetized by the U.S. government via the controversial Coinage Act of 1873 in part due to a surplus of the precious metal resulting from Comstock Lode mine production.
In all, nearly 7 million tons of silver and gold ore were extracted from the Comstock Lode over a period of just twenty years. From 1859 – 1878, production totaled $320 million, the modern-day equivalent of $9.6 billion.
The Typical Household Debt Mother Lode
The typical American household possesses an amount of debt reminiscent of the seemingly unending veins of ore in Virginia City’s Comstock Lode.
According to The Balance, the median mortgage size for homes purchased in 2017 was $211,500. At a 4% interest rate and 30-year term, that puts the average monthly mortgage payment at $1,009.73/month. This doesn’t include items such as property tax, home insurance, or PMI.
Meanwhile, the average new car loan balance in 2017 was $30,294, with a 69-month term. The 43% of Americans who have an auto loan are making an average monthly payment of $509 for new autos and $367 for those purchased used.
The average outstanding student loan balance is currently $26,700, while the the Federal Reserve reports that the average monthly payment now rings up at $393.
Finally, the 38.1% of households who carry credit card debt month-to-month do so with an average balance of $10,955. The national average interest rate of 16.41% and a middle-of-the road 3.5% minimum payment calculation puts the required monthly payment on this debt at $383.43.
Add up the typical mortgage, auto loan, student loan, and credit card debt from above and you have a grand total of $279,449 in common household debt.
There’s Gold In Them Thar Loans!

Virginia City is known as the birthplace of Mark Twain, as it was here that local newspaper reporter Samuel Clemens first used the famous pen name. The fact that Twain originally worked as a Comstock Lode miner before trading his pick-axe for a pen is an oft-overlooked historical nugget.
Mark Twain’s mining background shows in his use of the now-famous “There’s Gold In Them Thar Hills” mining expression in some of his writings. In a similar vein, there’s gold in them thar loans as well. The key is to start viewing your loans as sources of potential income and wealth, akin to the old Comstock Lode mines in their heyday.
Think of each of your loans as a gold mine. The required monthly payment for each loan represents the potential income you can re-claim by working that particular mine until the loan is paid off. The remaining interest you’ll pay over the life of each loan represents the vast store of potential wealth in each mine which you can claim for your own.
Extra payments constitute the pick-axe and shovel which you need to extract these riches. Like a golden glitter on the side of a mining shaft, this warrants a closer look.
Give Yourself A Raise By Working The Debt Gold Mine

The typical daily wage for a miner was $.25/day during the mid-1850’s. But the Comstock Lode mines of Virginia City paid a wage of $4/day, 16x the going rate. This gave miners the opportunity to cash in on the wealth of the Comstock Lode by boosting their income.
Your debt offers you a just as lucrative but far less dangerous means of effectively increasing your income. Consider the payments of the four common household debt line items referenced above:
- Mortgage Payment – $1,009.73/month, $12,116.76/year
- Auto Loan Payment – $438/month (average of new/used), $5,256/year
- Student Loan Payment – $393/month, $4,716/year
- Credit Card Payment – $383.43/month, $4,601.16/year
Eliminating even the smallest of the above payments translates to almost $400 per month of additional breathing room in our example household’s monthly budget.
Paying off your loans has the effect of increasing your discretionary income and the net profit of your monthly budget, even if you don’t earn a single penny more. This household can effectively give themselves a raise of $513.27/week, $2,224.16/month, or $26,689.92/year by eliminating their debt.
How much of a weekly, monthly, and yearly raise can you give yourself by eliminating the sum of the monthly payments on all of your loans?
Unlike your actual job, whether you receive such a raise is entirely up to you. Working the mines of the Debt Mother Lode can pay large dividends – all you need to do is put in the sweat needed to reach pay dirt.
Your Debt Gold Mine – A Lucrative Long-Term Investment
Increasing your discretionary income and the net profit of your monthly budget is only one benefit of mining your debt through the use of extra payments. The other holds the potential to pay off even more handsomely.
When you purchase something on credit with an interest rate greater than 0%, you allow the compound interest double agent to start working against you. And as if that wasn’t bad enough, there’s another, even larger danger of debt known as opportunity cost.
Like dynamite with a short fuse, compound interest makes debt something to avoid if at all possible. But if you already HAVE debt, it’s too late to simply avoid it. So rather than beating yourself up about the money you’re losing to interest, view your loans as money-making investments instead.
How much of the interest you have yet to pay on each of your loans can you avoid by paying the loan off ahead of schedule? This represents the potential earnings of each mine on your debt claim.
With each dollar of extra payments that you invest in working a given mine, you can expect a return. And unlike the risks and uncertainties of mining, when it comes to your loans your return is guaranteed.
A Debt Gold Mine Survey

The mining value of a given debt is determined by the amount of interest you have yet to pay over the remaining term of the loan, as well as the opportunity cost of that interest. Let’s use the four common household debt line items referenced in the Debt Mother Lode section above as an example:
- Mortgage – $211,500 balance, 4% interest, 30-year term, $1,009.73335/month
- Auto Loan – $30,294 balance, 5% interest, 69-month term, $509.00/month
- Student Loan – $26,700 balance, 6% interest, 84-month term, $393.00/month
- Credit Card Debt – $10,955 balance, 16.41% interest, $383.432/month
Using this cost of debt calculator and a 7% return on investments, we discover the potential value of each of these hypothetical debt mines is as follows:
- Mortgage – $802,166.38 (!!)
- Auto Loan – $10,623.25
- Student Loan – $14,359.83
- Credit Card Debt – $6,537.47
The total combined loan interest and opportunity cost of this debt is an incredible $833,686.93. While some might view this total with dismay, you should be rubbing your hands with ’49er glee. This value represents the amount of riches you can potentially claim by mining the debt in this example with extra payments!
Each extra payment made on this debt lowers the loan interest as well as the related opportunity cost of that interest. Think about it – NOT paying something you would otherwise have to is effectively the same as EARNING that same amount. This is where the concept of striking it rich by mining your debt comes into play. Are you seeing the gold in them thar loans yet?
A Credit Card Gold Mine
Let’s use the average credit card balance information from the Debt Mine Survey section above to illustrate the potential returns on extra payment investments in a credit card gold mine.
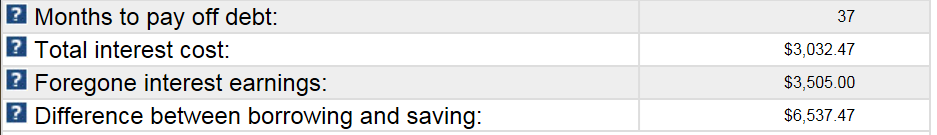
Using this cost of debt calculator, we find that if we simply make the required minimum payment of $383.432 on our $10,955 balance at 16.41% interest, we’ll pay off the balance in 37 months and pay $3,032.47 in interest and $3,505.00 in opportunity cost along the way, a total penalty of $6,537.47:
But an investment in the form of making an extra $100 payment every month reduces the payoff timeline by 9 months and drops the total of the interest and opportunity cost by $1,779.78 ($6,537.47 – $4,757.69):
In this example, your $2,800 investment in this credit card mine at a rate of $100/month over a period of 28 months earned a total return of $1,779.78. This represents 27.2% ($1,779.78 / $6,537.47) of the available precious metal in this mine which you were able to claim prior to the mine playing out. Working this debt mine with a higher rate of extra payments would result in an even higher recovery percentage!
A Mortgage Gold Mine
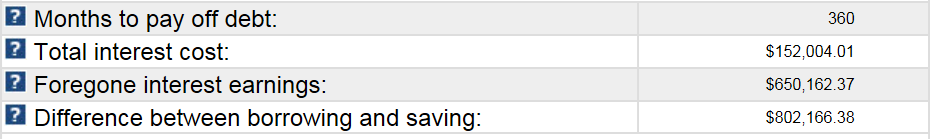
Let’s use the median new mortgage balance in 2017 of $211,500 over a period of 30 years at 4% interest to illustrate the potential returns on extra payment investments in a mortgage mine.
Using this cost of debt calculator, we find that if we simply make the required minimum payment of $1,009.73335 we’ll pay off the mortgage in 30 years and pay a total of $152,004.01 in interest and $650,162.37 in opportunity cost along the way, a total penalty of $802,166.38:
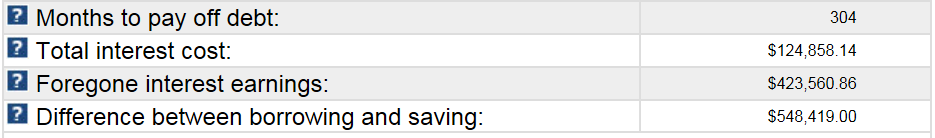
But an investment in the form of making an extra $100 payment every month cuts the payoff timeline down by 4 years and 8 months while reducing the total interest and opportunity cost by $253,747.38:
In this example, your $30,400 investment in this mortgage gold mine at a rate of $100/month over a period of 25 years and 4 months (304 payments) earned a total return of $253,747.38 ($802,166.38 – $548,419.00).
This represents 31.6% of the available precious metal which you were able to recover prior to the mine playing out. Working this debt mine with a higher rate of extra payments would result in a still higher recovery percentage!
Strike It Rich By Mining Your Debt

Mining your debt with extra payments can result in massive savings. It was this very concept that inspired Mrs. FFP and I to make quadruple payments on our own mortgage years ago, paying it off in just three years and two months start to finish.
Doing so enabled us to extract the vast majority of the wealth within our mortgage debt mine, saving thousands upon thousands of dollars in loan interest and opportunity cost. We’ve never regretted our decision to pay this loan off early, and it continues to pay dividends to this very day.
Use the previously discussed concepts of loan interest and opportunity cost to calculate the potential value of your personal Debt Mother Lode. If you feel strapped for cash, it’s time to stake your claim and grab that pick-axe so you too can strike it rich by mining your debt.
Staking Your Own Debt Claim
For those of you who have been following the Master Your Money series from the beginning, below are the next steps on your journey to financial freedom:
- Determine the size of your Debt Mother Lode by adding the balances of all your loans as listed on your Mint account overview.
- Calculate the size of the weekly, monthly, and yearly raise you can give yourself in the absence of debt by totaling the monthly payments for all of your loans as listed in Mint Budgets.
- Determine the potential wealth stored in each of your loans by using this cost of debt calculator and the principles of opportunity cost. You’ll need to know the interest rate, current balance, and monthly payment for each loan, which will all be available within Mint.
Extra Bonus – Click here for an entertaining recounting of life in historical Virginia City, as well as what the area looks and feels like today.




I’m on the home stretch of paying off my car loan. I threw an extra $500 last month and might throw an extra $500 or more this month as I have about 4k left and really want to get it off the books ASAP and open up that $450 in cash flow for other purposes!
Sounds like you’re getting awfully close to hitting pay dirt! That $950/mo. is going to make you feel rich once it’s no longer tied up in debt service. Keep swinging that pick-axe and filling those hypothetical mine carts with ore! You’ll never regret it, and your future self will thank you.
I like it! Although I was a little worried coming in you were going to talk about using leverage (where you take out a loan to invest), but this is much better.
Ye Gads! I can see how one could arrive at that impression given the title, but rest assured that is about as far from my approach as you can get. Talk about the dangers of dynamite. Fire in the hole!
I have read and got a lot of good information from all of your posts to date. However, I have an issue with your use of the cost of debt calculator in these examples. As I understand it, this calculator is based on paying cash for something, rather than taking out a loan. The calculator provides the interest avoided by paying cash and the opportunity income gained by investing the avoided interest payments. Your examples are for additional payments for a loan or credit card balance, which is not the same thing.
For the mortgage example the extra $100 principle payment each month does avoid about $27,000 in interest charges and results in the entire principle being paid off 4 years, 8 months early. However, none of the interest avoided along the way is available as cash flow to be invested. That said, the early payout does free up a monthly cash flow of $1,009.73 that would have been paid on the loan if the additional payments had not been made. Investing this cash flow for the 4 years, 8 months at 7% results in a total value of $66,646, which makes sense to me as the opportunity gain from making the additional payments on the loan.
I used this Calculator to determine this amount: https://keisan.casio.com/exec/system/1234231998
One other consideration for the mortgage example. If the $100/month had been invested at 7% for the 360 month term of the loan, rather than applying it to the loan principle, the ending value from the same calculator is $121,997.
What do you think?
Hello Jon, and thanks for the comment! I’m glad you’ve gotten some mileage out of my previous posts. And I appreciate hearing from people who have actually run the numbers for themselves rather than simply taking my word for it.
Your comment initially caused me some consternation, as all of your math made sense to me at a glance, yet clearly differed from the mortgage case study numbers in the article. I therefore made a point of revisiting this over the weekend to triple-check the math.
I believe your findings and mine are both technically correct, and that the difference is simply a matter of perspective. This took me a bit to wrap my mind around, but I’ll do my best to explain my thought process below.
You stated that your understanding of the cost of debt calculator is as follows:
To my mind, this a fair and accurate assessment. But there is an alternative way of interpreting the calculator and its intended use, which is also correct and differs only in perspective. The alternative definition would go something like this:
This is essentially the same definition that you provided, stated in reverse. I believe both statements are equally true, but from what I can tell the latter is the perspective which the author of the calculator used when building it, and it happens to be the lens through which I view the “cost of debt” concept as well.
I believe these two different perspectives can lead to two different methods of calculating opportunity cost. For example, the numbers you submitted using the calculator linked in your comment make perfect sense given the definition you provided:
Making $100/mo. extra payments from the mortgage case study results in a savings in loan interest paid of $27,145.87 ($152,004.01 – $124,858.14) over the life of the loan. And the $1,009.73 minimum payment invested at 7% for 4 years and 8 months (the 56 remaining payments which were avoided) totals another $66,646.36 in opportunity cost savings.
Together, this represents $93,792.23 in savings attributable to an investment of $30,400 (304 extra monthly payments of $100 each). While still impressive, this is significantly less than the savings of $253,747.38 illustrated by the calculator results in the article itself – hence your question.
Now let’s shift gears and view the cost of debt calculator and its results from the second perspective referenced earlier.
Of course, the amount of loan interest saved does not change, and remains at $27,145.87. But the opportunity cost savings calculation changes significantly.
Rather than calculating the savings based upon the $1,009.73/mo. payment invested at 7% for a duration of 4 years and 8 months ($66,646.36), the savings is calculated by subtracting the growth of the Foregone Future Value amount which would have otherwise occurred if it continued growing at 7% annually during the last 4 years and 8 months of the original 30-year loan term.
Effectively, the homeowner in the original case study is able to shut down the compound interest double agent after just 25 years and 4 months, rather than letting it run for the full 30 years.
Based on the cost of debt calculator results, we can see that the Foregone Future Value with no extra payments is $650,162.37. But eliminating the ability of compound interest to continue working against the homeowner for the last 4 years and 8 months reduces this amount to $423,560.86.
That’s a difference of $226,601.51, which when added to the $27,145.87 in loan interest saved results in the $253,747.38 figure from the article.
Due to the nature of compound interest, the growth of a balance over a 4 year and 8 month time horizon differs significantly based on whether one looks at the first five year window or looks at a such a window after 25 years have elapsed.
Looking at the tables generated from the cost of debt calculator for the original and extra payment scenario, you’ll note that the Foregone Interest amount after payment #304 in the scenario without extra payments starts at $2,696.10/month and ends at $3,770.62/month.
This is a significant difference from the $4.11/month – $260.66/month in Foregone Future Interest accrued over the course of the first 56 payments.
For sake of illustration, use your above calculator to take the bonus 4 year and 8 month period in which that $1,009.73/month mortgage payment is freed up to be invested and expand it to a 30 year time horizon with 7% annual return. Then instead of looking at the balance accrued after the first 4 years and 8 months of that time horizon ($66,646.36), look at the growth in the balance over the last 4 years and 8 months of those 30 years – $390,559.99 ($1,231,841.31 – 841,281.32).
Effectively, the additional 4 years and 8 months with which this money can be invested lengthens the available time horizon over which it can be grown with compound interest.
This was an eye-opener for me years ago when we were formulating plans regarding whether to pay off our mortgage prior to saving aggressively for retirement via 401K plan contributions or vice versa.
Delaying the start of our contributions to our 401K by the duration of our mortgage payoff timeline (3 years and 2 months) at first didn’t seem as if it would be a big deal, because the accumulated investment growth over those 3 years and 2 months was relatively paltry. But that picture drastically changed once I understood that the opportunity cost of delaying the start of investing by 3+ years was effectively the same as shortening my overall investment time horizon by three years.
The proper way to calculate the true cost of delaying investing is to look at the hypothetical growth over the time period considered at the END of your investment time horizon. Paints a very different picture!
Last but not least, you make an astute observation regarding the $121,997 that could have been earned if that same $100/mo. extra payment had been invested at 7% rather than used to make extra payments on the mortgage loan at 4%.
The mortgage vs. invest debate has many different facets, a topic best saved for another day. That said, unless the loan in question has an interest rate greater than 7%, it’s generally more efficient to invest excess cash in the stock market than it is in the loan. This of course entails the risk of building equity aggressively in a mortgage only to lose a job or otherwise find yourself limited in cash flow and unable to make minimum payments, resulting in repossession. And there’s a host of other emotional and psychological factors at play as well.
I am planning a future article in which I detail why it was actually more mathematically efficient in our situation to pay down the mortgage prior to investing in the stock market, even though our mortgage rate was significantly less than 7%.
Thank you for your comment, and I hope this response makes sense!
Thank you for the detailed and well thought out response to my comment. I agree with most of what you have said, but cannot agree with the way you are using the Cost of Debt calculator to calculate savings.
As I see it, the calculator is useful in comparing two mutually exclusive options:
1. Taking out a loan and paying interest to the bank, thus reducing future net worth by the total amount of interest paid, or
2. Paying cash for the purchase and investing the monthly interest amounts that would have otherwise gone to the bank to increase future net worth over the same period as the loan.
For large loans, such as used in the house purchase example, the difference between paying interest on a loan vs. buying with cash and investing the monthly interest that would have otherwise been paid produces truly eye opening differences in future net worth.
However, once option 1 (the loan) is chosen, option 2 to invest the interest is no longer a possibility since the monthly interest amounts are fully consumed by the monthly loan payments. We cannot simultaneously use the money to pay the loan interest and also invest it to create the Foregone Interest Earnings amount that is generated by the calculator. In other words, the actual Foregone Interest Earnings presented by the calculator must be considered as $0.00 once the loan is signed. By signing the loan we gave up option 2 – it is never funded and the value provided by the calculator for Foregone Interest Earnings is void.
The same logic applies to the situation where the borrower chooses to make additional principle payments. These payments have the effect of reducing both the total interest paid and also the term of the loan. Obviously, additional payments can only be made if option 1 (the loan) is chosen in the first place (i.e. there can be no additional payments if there is no loan). In this scenario the reduced monthly interest amounts resulting from the additional payments are also fully consumed by the loan payments so the actual Foregone Interest Earnings must also be considered as $0.00. In the home loan example from your post the only real savings achieved by making additional payments is the interest reduction of $27,145.87. No additional “savings” can be determined by comparing the two Foregone Interest Earnings from the Cost of Debt calculator because those numbers do not represent the reality that the loan was the choice made. The $223,601.51 savings you are claiming is not real.
In your reply to my earlier comment you start a paragraph “Looking at the tables generated from the cost of debt calculator….” These tables are only valid if there is no loan. The tables are based on investing the monthly interest that would have otherwise been paid to the bank to satisfy the loan. Since we are dealing with two scenarios where there actually is a loan there is no money to invest and the tables have no data. Once the decision is made to take out the loan, the Interest Earnings are truly Forgone, aka. $0.00.
As I indicated in my earlier comment, the borrower must wait until the early payoff of the loan is completed before the cash flow from the loan payments becomes available to invest (for the 4 years, 8 months to complete the original 360 month loan period in the example). None of the monthly interest reduction is available to invest each of the previous 25 years, 4 months as those amounts go to reduce the outstanding balance of the loan. In your reply you also incorrectly added the $27,145.87 interest savings to the $66,646.36 that resulted from investing the last 56 loan payment amounts. This is not correct because $22,103.77 of the interest savings actually went toward reducing the outstanding balance of the loan over the 304 month payment period. The remaining $5,042.10 of interest savings comes from the interest that that wasn’t paid on the last 56 payments of the 360 month loan. These interest savings became a part of the $1,009.73 that was invested over 56 months to generate the $66,646.36. I was able to determine where the interest savings went by using the calculator in the next paragraph and copying the resulting data into a spreadsheet.
The additional payments resulted in “mining” a benefit of $66,646.36 by the end of the 360 month period of the original loan. This is a great head start on future investment returns vs. waiting the additional 56 months for the $1,009.73 to become available for investment. If we continue investing the $1,009.73 monthly for an additional 20 years the $66,646.36 becomes $795,162 vs. $525,995 for the later start. That $269,167 difference is a really nice pile of gold resulting from mining this loan with additional payments!
As I see it, the Cost of Debt calculator is a valuable tool for understanding the full cost of using debt vs. saving to pay cash for something. It could also be used for evaluating the benefit of buying a used car for cash vs. a new car with a loan, or buying a less expensive home and investing the difference in monthly interest payments compared to the loan on a more expensive home. Once the choice is made to take out a loan, the usefulness of the Cost of Debt calculator is over. From then on a calculator such as this one https://www.bankrate.com/calculators/home-equity/additional-mortgage-payment-calculator.aspx would provide less confusing information on the benefit of making additional payments.